Browse By Unit
1.4 Estimating Limit Values from Tables
2 min read•june 18, 2024
So far, we’ve defined limits, used limit notation, and estimated limit values from graphs. In this study guide, we’ll cover how to estimate limit values from tables given to you. 🎯
Concept of a Limit Recap
As our previous key topic guides have mentioned, a limit is the value at which x is near the target number a is defined. It is typically written like the example below:
Here, we see that the arrow indicates that x is approaching the target number a and L represents the limit of the function when x is close or near the target number a. There are several ways of finding a limit for a function, and one of these ways will be discussed in the following section. 🔢
One-Sided Limits
Before we begin, it is also important to understand the concept of one-sided limits, where the limit is defined as x approaching the target number, a, from either the left or right side of the number line. You could think of this as the left side going from numbers less than the target number and the right side going from numbers greater than the target number.
Whether or not a limit is coming from the left or right side of the target value is indicated using a plus sign (+), meaning it approaches from the right ⬅️, or a minus sign (-), meaning it approaches from the left ➡️, at the top corner of the target value
Using a Table to Estimate Limits
In some cases, direct substitution of the target number does not work in solving for a limit of a function because it leads to an indeterminable type (which is a topic discussed later in the course). ❌ Essentially, you cannot substitute the target value directly into the function because the output will be undefined, as the example below displays:
Here we see that if we try to use the direct substitution method by plugging in 0 into the function, we get , which is undefined and implies that the limit of the function at 0 is also undefined.
So to solve for the limit of these particular functions, x-values close to the target number a from both the left and right sides of the number line can be substituted into the function. In doing so, the outputs from both sides of the target number will approach a certain output value, which would be considered the limit of the function at the target number a. ↔️
To make it more organized, we use a table to list the values less than or greater than the target number in the top row and list the limit of the function at those values on the next row. An example is displayed below for better understanding. 📚

Image courtesy of Calculus: 9th Edition by Larson and Edward.
This table results from substituting x-values near 0 from the left and right sides in the function mentioned above and calculating their outputs 📱. As displayed in the table…
- As the x-values get closer to 0 from the right side, the y-values are getting closer to 2.
- As the x-values from the left side get closer to 0, the y-values are also getting closer to 2, but the y-values of this function never actually reach 2.
Based on the movement of the y-values as the x-values approach 0, we can estimate that the limit of the function at x = 0 would be 2.
Keep in mind that for this method to work, the limit from the left AND right side relative to the target number has to be approaching the SAME output value, as the example above shows.
In cases where this method does not work, such as the limit of the function from the left and right sides of the target number a are not approaching the same y-value, it could imply that a vertical asymptote exists in this function at the target number, which will be explained in future key topic guides throughout the unit. 📈
Practice Evaluating Limits from a Table
Question courtesy of Calculus 9th Edition by Larson and Edward textbook.
To begin solving for the limit, a table of x-values that are greater and less than 4 needs to be made.
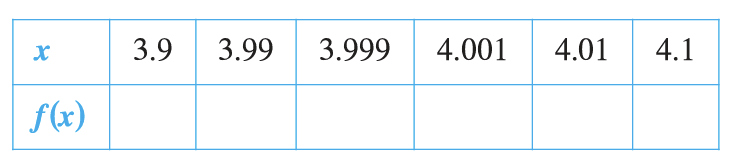
Image courtesy of Calculus 9th Edition by Larson and Edward.
Now that we have the table made, we can plug each x-value into the function to find the y-value that is being approached from the left and right sides.
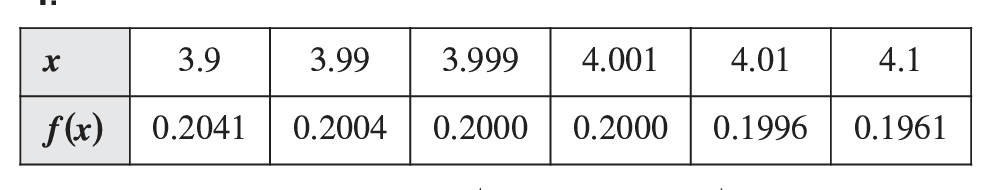
Image courtesy of Calculus 9th Edition by Larson and Edward
By calculating the y-value for each x-value near 4 from the left and right sides, we can estimate that 0.2 is the limit of the function as x approaches 4 because both the x-values from both left and right sides of the target number 4 are approaching the same y-value, which is 0.2. 💾
Conclusion
Using a table is one of many ways to solve for limits that cannot be solved through direct substitution. While tedious, it can provide insight into the direction of the y-values when approaching a given x-value, which is critical in solving for limits. Thanks for reading and good luck! ✅
<< Hide Menu
1.4 Estimating Limit Values from Tables
2 min read•june 18, 2024
So far, we’ve defined limits, used limit notation, and estimated limit values from graphs. In this study guide, we’ll cover how to estimate limit values from tables given to you. 🎯
Concept of a Limit Recap
As our previous key topic guides have mentioned, a limit is the value at which x is near the target number a is defined. It is typically written like the example below:
Here, we see that the arrow indicates that x is approaching the target number a and L represents the limit of the function when x is close or near the target number a. There are several ways of finding a limit for a function, and one of these ways will be discussed in the following section. 🔢
One-Sided Limits
Before we begin, it is also important to understand the concept of one-sided limits, where the limit is defined as x approaching the target number, a, from either the left or right side of the number line. You could think of this as the left side going from numbers less than the target number and the right side going from numbers greater than the target number.
Whether or not a limit is coming from the left or right side of the target value is indicated using a plus sign (+), meaning it approaches from the right ⬅️, or a minus sign (-), meaning it approaches from the left ➡️, at the top corner of the target value
Using a Table to Estimate Limits
In some cases, direct substitution of the target number does not work in solving for a limit of a function because it leads to an indeterminable type (which is a topic discussed later in the course). ❌ Essentially, you cannot substitute the target value directly into the function because the output will be undefined, as the example below displays:
Here we see that if we try to use the direct substitution method by plugging in 0 into the function, we get , which is undefined and implies that the limit of the function at 0 is also undefined.
So to solve for the limit of these particular functions, x-values close to the target number a from both the left and right sides of the number line can be substituted into the function. In doing so, the outputs from both sides of the target number will approach a certain output value, which would be considered the limit of the function at the target number a. ↔️
To make it more organized, we use a table to list the values less than or greater than the target number in the top row and list the limit of the function at those values on the next row. An example is displayed below for better understanding. 📚

Image courtesy of Calculus: 9th Edition by Larson and Edward.
This table results from substituting x-values near 0 from the left and right sides in the function mentioned above and calculating their outputs 📱. As displayed in the table…
- As the x-values get closer to 0 from the right side, the y-values are getting closer to 2.
- As the x-values from the left side get closer to 0, the y-values are also getting closer to 2, but the y-values of this function never actually reach 2.
Based on the movement of the y-values as the x-values approach 0, we can estimate that the limit of the function at x = 0 would be 2.
Keep in mind that for this method to work, the limit from the left AND right side relative to the target number has to be approaching the SAME output value, as the example above shows.
In cases where this method does not work, such as the limit of the function from the left and right sides of the target number a are not approaching the same y-value, it could imply that a vertical asymptote exists in this function at the target number, which will be explained in future key topic guides throughout the unit. 📈
Practice Evaluating Limits from a Table
Question courtesy of Calculus 9th Edition by Larson and Edward textbook.
To begin solving for the limit, a table of x-values that are greater and less than 4 needs to be made.

Image courtesy of Calculus 9th Edition by Larson and Edward.
Now that we have the table made, we can plug each x-value into the function to find the y-value that is being approached from the left and right sides.

Image courtesy of Calculus 9th Edition by Larson and Edward
By calculating the y-value for each x-value near 4 from the left and right sides, we can estimate that 0.2 is the limit of the function as x approaches 4 because both the x-values from both left and right sides of the target number 4 are approaching the same y-value, which is 0.2. 💾
Conclusion
Using a table is one of many ways to solve for limits that cannot be solved through direct substitution. While tedious, it can provide insight into the direction of the y-values when approaching a given x-value, which is critical in solving for limits. Thanks for reading and good luck! ✅

© 2024 Fiveable Inc. All rights reserved.