Browse By Unit
Dylan Black
Dalia Savy
Dylan Black
Dalia Savy
Review of Reversible Reactions
Like we discussed in the last study guide, equilibrium can be described as the settling of the rates of reversible reactions. These are reactions that can go forward (A → B) and backward (B → A) to form one full equilibrium reaction: A ⇌ B.
Equilibrium is the point at which the rates of these reactions equal, implying that the amount of A created is equal to the amount of B created over a period of time meaning that their concentrations do not change and the reaction mixture stays constant. We can represent this graphically by showing both the rates of reaction and the concentrations of the products and reactants as the reaction goes forward:
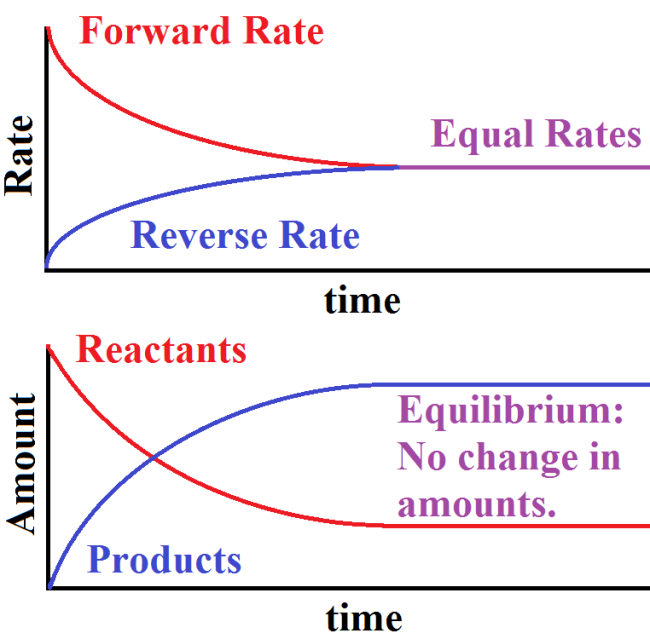
As we can see, when looking at a graph of rates, the forward reaction starts off as the reaction happening fastest but the reverse rate eventually catches up until the two are at equal rates.
This can be seen further by looking at the number of products and reactants present as the reaction goes forward. At first, we see an accumulation of products because the forward rate exceeds the reverse rate implying a net creation of products. However, once equilibrium is reached, these values do not change and are constant. The reactions continue proceeding, just at equal rates.
Favored Direction of Reaction
When discussing equilibrium, a topic that comes up a lot is whether a reaction is “product-favored” or “reactant-favored.” This concept describes whether a reaction goes forward more than backward or backward more than forward. The main question is: which direction is favored? The direction that is favored is going to proceed more.
In the picture above, we can see that this reaction is product favored because, at equilibrium, the concentration of products is larger than the concentrations of reactants. This can be represented mathematically as well by looking at values of K for a reaction.
- If K < 1, the reaction is reactant-favored (some K values can get into the range of 10^(-50)!)
- If K > 1, the reaction is product-favored (for some reactions K values are so large we say the reaction goes entirely forward)
- If K = 1, we have an equal proportion of reactants and products at equilibrium. This is the least likely result.
Conceptually Understanding This
Take a look at these examples of product-favored and reactant-favored reactions:
N₂O₄ ⇌ 2NO₂ ⇒ K = 4.65 * 10⁻³.
Because K < 1, this reaction is reactant-favored. The easiest way to think of this is to go back to what K means! K is generally the ratio of the products over the reactants. Therefore, if the reactants are favored and in greater quantity, the denominator of K is going to be larger than the numerator. The smaller the denominator, the smaller K is. This is one way to explain why the reaction is reactant favored when K < 1.
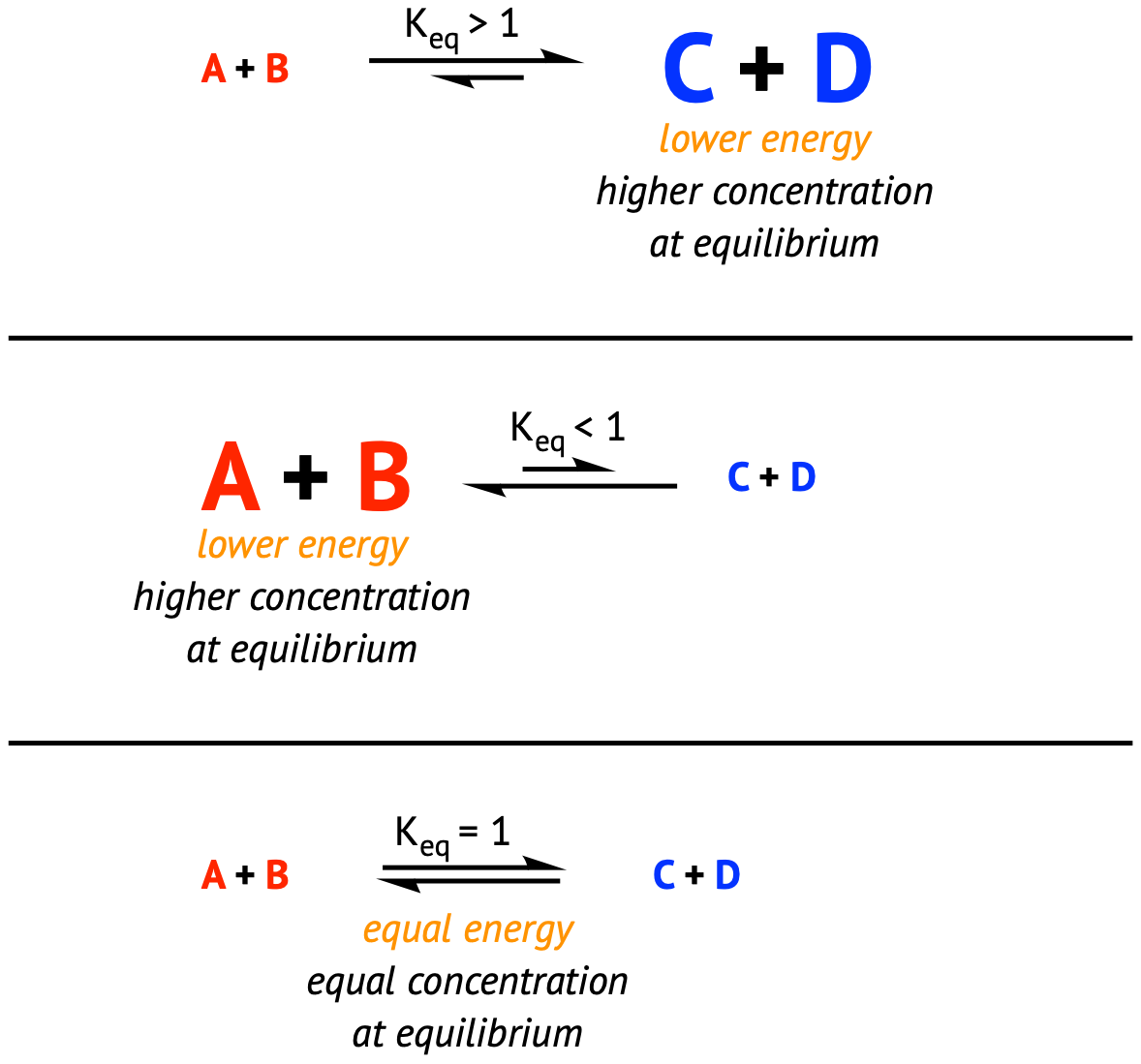
Image Courtesy of AlChemie
This works the other way too!
2O₃ ⇌ 3O₂ ⇒ K = 2.5 * 10¹₂.
Because K > 1, this reaction is product-favored. Since there are more products here, the numerator of K is going to be larger than the denominator. The larger the numerator, the larger K is.
What Does A Reaction’s “Direction” Tell Us?
By observing whether a reaction is product-favored or reactant-favored, we are able to draw some incredibly useful conclusions about how the reaction is going to proceed. It’s worth remembering that all reactions will produce some products and K tells us how much product is actually created. By observing K, we can make qualitative judgments on how far forward a reaction goes.
A higher K value implies that from reactants, more products are created. For example, if we have two reactions and one has a K of 1.310⁶ and the other 2.310⁸, we know that both of these reactions are product favored but which one is more product favored? From observing the K values of each of these reactions, we see that the second reaction will go farther forward than the first. In a similar vein, we know that a reaction with a lower K value will not go as far forward.
Acid-Base Example
This way of thinking about K values proves incredibly useful, especially when applying equilibrium to real-life scenarios such as acids and bases. For example, consider acetic acid versus carbonic acid.
For acetic acid, the reaction CH₃COOH ⇌ CH₃COO⁻ + H⁺ has a K value of 1.8 * 10⁻⁵, whereas for carbonic acid the reaction H₂CO₃ ⇌ H⁺ + HCO₃⁻ has a K value of 4.3 * 10⁻⁷. From this information, we can make a few useful conclusions.
We see that acetic acid has a higher dissociation constant (K value), meaning it will go further forward than carbonic acid even though both are reactant-favored. This tells us that with equal concentrations of acetic acid and carbonic acid, acetic acid will produce more H+ ions than H₂CO₃. From there, we can evaluate that acetic acid is in fact a stronger acid than carbonic acid (at the same concentration).
Conclusion
This section stands out as one of the most important conceptual sections of unit seven. In future study guides, the direction of a reversible reaction will be used to justify mathematical calculations and the ways that a reaction may react to external changes in pressure, concentration, and temperature. In fact, in the next section, we’ll dive into one of these topics with our discussion of Q, the reaction quotient. By understanding this section in depth the mathematical calculations of unit seven will be infinitely easier.
<< Hide Menu
Dylan Black
Dalia Savy
Dylan Black
Dalia Savy
Review of Reversible Reactions
Like we discussed in the last study guide, equilibrium can be described as the settling of the rates of reversible reactions. These are reactions that can go forward (A → B) and backward (B → A) to form one full equilibrium reaction: A ⇌ B.
Equilibrium is the point at which the rates of these reactions equal, implying that the amount of A created is equal to the amount of B created over a period of time meaning that their concentrations do not change and the reaction mixture stays constant. We can represent this graphically by showing both the rates of reaction and the concentrations of the products and reactants as the reaction goes forward:

As we can see, when looking at a graph of rates, the forward reaction starts off as the reaction happening fastest but the reverse rate eventually catches up until the two are at equal rates.
This can be seen further by looking at the number of products and reactants present as the reaction goes forward. At first, we see an accumulation of products because the forward rate exceeds the reverse rate implying a net creation of products. However, once equilibrium is reached, these values do not change and are constant. The reactions continue proceeding, just at equal rates.
Favored Direction of Reaction
When discussing equilibrium, a topic that comes up a lot is whether a reaction is “product-favored” or “reactant-favored.” This concept describes whether a reaction goes forward more than backward or backward more than forward. The main question is: which direction is favored? The direction that is favored is going to proceed more.
In the picture above, we can see that this reaction is product favored because, at equilibrium, the concentration of products is larger than the concentrations of reactants. This can be represented mathematically as well by looking at values of K for a reaction.
- If K < 1, the reaction is reactant-favored (some K values can get into the range of 10^(-50)!)
- If K > 1, the reaction is product-favored (for some reactions K values are so large we say the reaction goes entirely forward)
- If K = 1, we have an equal proportion of reactants and products at equilibrium. This is the least likely result.
Conceptually Understanding This
Take a look at these examples of product-favored and reactant-favored reactions:
N₂O₄ ⇌ 2NO₂ ⇒ K = 4.65 * 10⁻³.
Because K < 1, this reaction is reactant-favored. The easiest way to think of this is to go back to what K means! K is generally the ratio of the products over the reactants. Therefore, if the reactants are favored and in greater quantity, the denominator of K is going to be larger than the numerator. The smaller the denominator, the smaller K is. This is one way to explain why the reaction is reactant favored when K < 1.

Image Courtesy of AlChemie
This works the other way too!
2O₃ ⇌ 3O₂ ⇒ K = 2.5 * 10¹₂.
Because K > 1, this reaction is product-favored. Since there are more products here, the numerator of K is going to be larger than the denominator. The larger the numerator, the larger K is.
What Does A Reaction’s “Direction” Tell Us?
By observing whether a reaction is product-favored or reactant-favored, we are able to draw some incredibly useful conclusions about how the reaction is going to proceed. It’s worth remembering that all reactions will produce some products and K tells us how much product is actually created. By observing K, we can make qualitative judgments on how far forward a reaction goes.
A higher K value implies that from reactants, more products are created. For example, if we have two reactions and one has a K of 1.310⁶ and the other 2.310⁸, we know that both of these reactions are product favored but which one is more product favored? From observing the K values of each of these reactions, we see that the second reaction will go farther forward than the first. In a similar vein, we know that a reaction with a lower K value will not go as far forward.
Acid-Base Example
This way of thinking about K values proves incredibly useful, especially when applying equilibrium to real-life scenarios such as acids and bases. For example, consider acetic acid versus carbonic acid.
For acetic acid, the reaction CH₃COOH ⇌ CH₃COO⁻ + H⁺ has a K value of 1.8 * 10⁻⁵, whereas for carbonic acid the reaction H₂CO₃ ⇌ H⁺ + HCO₃⁻ has a K value of 4.3 * 10⁻⁷. From this information, we can make a few useful conclusions.
We see that acetic acid has a higher dissociation constant (K value), meaning it will go further forward than carbonic acid even though both are reactant-favored. This tells us that with equal concentrations of acetic acid and carbonic acid, acetic acid will produce more H+ ions than H₂CO₃. From there, we can evaluate that acetic acid is in fact a stronger acid than carbonic acid (at the same concentration).
Conclusion
This section stands out as one of the most important conceptual sections of unit seven. In future study guides, the direction of a reversible reaction will be used to justify mathematical calculations and the ways that a reaction may react to external changes in pressure, concentration, and temperature. In fact, in the next section, we’ll dive into one of these topics with our discussion of Q, the reaction quotient. By understanding this section in depth the mathematical calculations of unit seven will be infinitely easier.

© 2025 Fiveable Inc. All rights reserved.