Browse By Unit
Daniella Garcia-Loos
Saarah Hasan
Daniella Garcia-Loos
Saarah Hasan
We've all heard of Einstein's famous equation, E=(Δm)c^2. In this lesson, we'll be going over how it can be used to calculate the mass equivalent for a given amount of energy transfer or an energy equivalent for a given amount of mass change.
Binding Energy
Binding energy is the energy required to separate the particles in a system from each other. It is a measure of the strength of the forces that hold the particles together.
Here are some key points about binding energy:
- Binding energy is the energy required to separate the particles in a system from each other. It is a measure of the strength of the forces that hold the particles together.
- The binding energy of a system is equal to the energy required to break the system into its individual particles. For example, the binding energy of a nucleus is equal to the energy required to separate the protons and neutrons in the nucleus.
- The binding energy of a system can be calculated using the equation BE = Etotal - Ei, where BE is the binding energy, Etotal is the total energy of the system, and Ei is the energy of the individual particles.
- The binding energy of a system can be positive, negative, or zero. A positive binding energy indicates that energy is required to separate the particles, while a negative binding energy indicates that energy is released when the particles are separated. A zero binding energy indicates that the particles are already separated and no energy is required to break them apart.
- The binding energy of a system is a measure of the stability of the system. Systems with high binding energy are more stable and less likely to break apart, while systems with low binding energy are less stable and more likely to break apart.
Since atoms are so, so small, we need small units. We use the atomic mass unit (amu/u), which is defined as 1/12 the mass of a carbon-12 nucleus. 1u=1.6605∗10−^22^7 kg7kg. m_p=1.00728u
m_n=1.00867u
Let’s look at the helium-4 nucleus; it contains 2 protons and 2 neutrons-the predicted would be 4.0330 u. But the experimental mass of the helium-4 nucleus is 4.0026 u: this difference of 0.0304 u is called the mass defect and is proportional to the binding energy of the nucleus (E=mc^2).
Einstein’s special theory of relativity tells us that the mass can be converted to energy; the energy equivalent of 1 u=931.5 MeV. The binding energy of any nucleus is therefore determined by the relationship: BE(MeV) = Mass Defect * 931.5 MeV/uBE(MeV)=MassDefect∗931.5MeV/u Avg BE= BE/A AvgBE=BE/A where AA = the mass number of the element
The greater the average binding energy, the greater the stability of the nucleus.
Some information on fission and fusion:
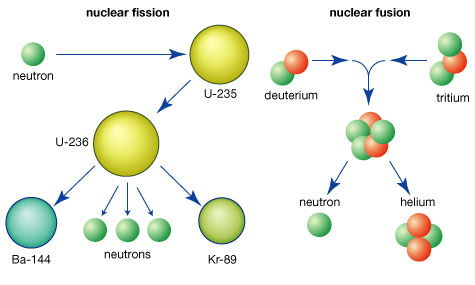
- Fission occurs when a heavy unstable nuclei splits into 2 smaller daughter nuclei by the absorption of a slow-moving neutron.
- Fusion occurs when two light nuclei fuse into a more stable isotope; unlike fission, fusion doesn’t produce radioactive by-products. Fission and fusion are two processes that release energy by changing the structure of atomic nuclei. They are both nuclear reactions and are used to generate electricity and for other applications.
Here are some key points about fission and fusion:
- Fission is a nuclear reaction in which an atomic nucleus is split into two or more smaller nuclei. It is a process that releases energy by changing the structure of the nucleus.
- Fusion is a nuclear reaction in which two or more atomic nuclei combine to form a larger nucleus. It is a process that releases energy by changing the structure of the nucleus.
- Fission and fusion are both nuclear reactions that release energy by changing the structure of atomic nuclei. They are both used to generate electricity and for other applications.
- Fission is a process that occurs naturally in some radioactive isotopes, but it can also be induced artificially by bombarding the nucleus with neutrons. It is used to generate electricity in nuclear power plants.
- Fusion is a process that occurs naturally in stars, but it can also be induced artificially in laboratories. It is used to generate electricity in fusion power plants.
Taken from Wikimedia Commons
Practice Problems:🧩
- Correct statements about the binding energy of a nucleus include which of the following?I. It is the energy needed to separate the nucleus into its individual protons and neutrons.II. It is the energy liberated when the nucleus is formed from the original nucleons.III. It is the energy equivalent of the apparent loss of mass of its nucleon constituents.
(A) I only (B) III only (C) I and II only (D) II and III only (E) I, II, and III
Answer:
- **E :**These are all true statements about binding energy
<< Hide Menu
Daniella Garcia-Loos
Saarah Hasan
Daniella Garcia-Loos
Saarah Hasan
We've all heard of Einstein's famous equation, E=(Δm)c^2. In this lesson, we'll be going over how it can be used to calculate the mass equivalent for a given amount of energy transfer or an energy equivalent for a given amount of mass change.
Binding Energy
Binding energy is the energy required to separate the particles in a system from each other. It is a measure of the strength of the forces that hold the particles together.
Here are some key points about binding energy:
- Binding energy is the energy required to separate the particles in a system from each other. It is a measure of the strength of the forces that hold the particles together.
- The binding energy of a system is equal to the energy required to break the system into its individual particles. For example, the binding energy of a nucleus is equal to the energy required to separate the protons and neutrons in the nucleus.
- The binding energy of a system can be calculated using the equation BE = Etotal - Ei, where BE is the binding energy, Etotal is the total energy of the system, and Ei is the energy of the individual particles.
- The binding energy of a system can be positive, negative, or zero. A positive binding energy indicates that energy is required to separate the particles, while a negative binding energy indicates that energy is released when the particles are separated. A zero binding energy indicates that the particles are already separated and no energy is required to break them apart.
- The binding energy of a system is a measure of the stability of the system. Systems with high binding energy are more stable and less likely to break apart, while systems with low binding energy are less stable and more likely to break apart.
Since atoms are so, so small, we need small units. We use the atomic mass unit (amu/u), which is defined as 1/12 the mass of a carbon-12 nucleus. 1u=1.6605∗10−^22^7 kg7kg. m_p=1.00728u
m_n=1.00867u
Let’s look at the helium-4 nucleus; it contains 2 protons and 2 neutrons-the predicted would be 4.0330 u. But the experimental mass of the helium-4 nucleus is 4.0026 u: this difference of 0.0304 u is called the mass defect and is proportional to the binding energy of the nucleus (E=mc^2).
Einstein’s special theory of relativity tells us that the mass can be converted to energy; the energy equivalent of 1 u=931.5 MeV. The binding energy of any nucleus is therefore determined by the relationship: BE(MeV) = Mass Defect * 931.5 MeV/uBE(MeV)=MassDefect∗931.5MeV/u Avg BE= BE/A AvgBE=BE/A where AA = the mass number of the element
The greater the average binding energy, the greater the stability of the nucleus.
Some information on fission and fusion:
- Fission occurs when a heavy unstable nuclei splits into 2 smaller daughter nuclei by the absorption of a slow-moving neutron.
- Fusion occurs when two light nuclei fuse into a more stable isotope; unlike fission, fusion doesn’t produce radioactive by-products. Fission and fusion are two processes that release energy by changing the structure of atomic nuclei. They are both nuclear reactions and are used to generate electricity and for other applications.
Here are some key points about fission and fusion:
- Fission is a nuclear reaction in which an atomic nucleus is split into two or more smaller nuclei. It is a process that releases energy by changing the structure of the nucleus.
- Fusion is a nuclear reaction in which two or more atomic nuclei combine to form a larger nucleus. It is a process that releases energy by changing the structure of the nucleus.
- Fission and fusion are both nuclear reactions that release energy by changing the structure of atomic nuclei. They are both used to generate electricity and for other applications.
- Fission is a process that occurs naturally in some radioactive isotopes, but it can also be induced artificially by bombarding the nucleus with neutrons. It is used to generate electricity in nuclear power plants.
- Fusion is a process that occurs naturally in stars, but it can also be induced artificially in laboratories. It is used to generate electricity in fusion power plants.

Taken from Wikimedia Commons
Practice Problems:🧩
- Correct statements about the binding energy of a nucleus include which of the following?I. It is the energy needed to separate the nucleus into its individual protons and neutrons.II. It is the energy liberated when the nucleus is formed from the original nucleons.III. It is the energy equivalent of the apparent loss of mass of its nucleon constituents.
(A) I only (B) III only (C) I and II only (D) II and III only (E) I, II, and III
Answer:
- **E :**These are all true statements about binding energy

© 2024 Fiveable Inc. All rights reserved.